Physicists solve uncertainty about uncertainty principle
September 10, 2012
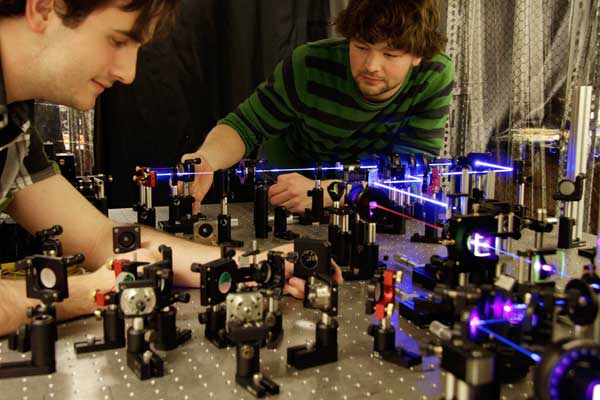
Taking on Heisenberg: Quantum optics graduate students Dylan Mahler (l) and Lee Rozema (r) prepare pairs of entangled photons to study the disturbance the photons experience after they are measured. Kids, don’t try this at home without special googles! (Credit: Dylan Mahler)
The Heisenberg uncertainty principle is one of the cornerstones of quantum mechanics: it’s impossible to measure anything without disturbing it.
For instance, any attempt to measure a particle’s position must change its momentum.
But never mind all that. It’s wrong, University of Toronto physicists say they have just proven.
(This has important implications for quantum information and especially quantum cryptography, where it is fundamental to the security of certain protocols.)
E pur non si muove
Here’s how PhD candidate Lee Rozema states the problem: “We designed an apparatus to measure a property — the polarization — of a single photon. We then needed to measure how much that apparatus disturbed that photo. To do this, we would need to measure the photon before the apparatus — but that measurement would also disturb the photon.”
To overcome this well-known impasse, Rozema and his colleagues used a technique known as “weak measurement” in which the effect of the measuring device is itself too weak to measure. Here’s how it worked:

Left: weak measurement of X or Z; center: strong measurement to determine Z; right: verification measurement of X (credit: L. Rozema et al.)
1. Before each photon is sent to the standard measurement apparatus, measure two forms of polarization, X and Z, weakly, then measure the Z polarization (strongly), and then measure the X polarization again (right), noting the effect of the Z measurement on the X polarization.
2 Measure it again, with the same setup, noting the results.
3. Repeat many times and compare the measurements.
Result: the disturbance induced by the measurement of Z was in fact less than Heisenberg’s precision-disturbance relation would require. (Actually, it was more complicated than that — see the open-access ArXiv paper for details.)
The findings build on recent challenges to Heisenberg’s principle by scientists the world over. Nagoya University physicist Masanao Ozawa suggested in 2003 that Heisenberg’s uncertainty principle does not apply to measurement, but could only suggest an indirect way to confirm his predictions. A validation of the sort he proposed was carried out last year by Yuji Hasegawa’s group at the Vienna University of Technology.
In 2010, Griffith University scientists Austin Lund and Howard Wiseman showed that weak measurements could be used to characterize the process of measuring a quantum system. However, there were still hurdles to clear as their idea effectively required a small quantum computer, which is difficult to build.
“In the past, we have worked experimentally both on implementing weak measurements, and using a technique called ‘cluster state quantum computing’ to simplify building quantum computers. The combination of these two ideas led to the realization that there was a way to implement Lund and Wiseman’s ideas in the lab,” says Rozema.
Implications
“The results force us to adjust our view of exactly what limits quantum mechanics places on measurement,” says Rozema. “These limits are important to fundamental quantum mechanics and also central in developing ‘quantum cryptography’ technology, which relies on the uncertainty principle to guarantee that any eavesdropper would be detected due to the disturbance caused by her measurements.
“The quantum world is still full of uncertainty, but at least our attempts to look at it don’t have to add as much uncertainty as we used to think!”
The research is supported by funding from Natural Sciences and Engineering Research Council of Canada and the Canadian Institute for Advanced Research.